Boundary conditions at interfaces between different media

Now we think a interface which is the boundary medium 1 and 2 as shown in Fig.1. From Gauss law, we can get the following for the Gauss box which include the interface inside the box,

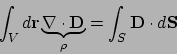$$\int_V d{\bf r} \underbrace{\nabla\cdot{\bf D}}_{\rho} = \int_S {\bf D}\cdot d{\bf S}$$ (38)

In the limit that the Gauss box is very thin

$$\int_V d{\bf r} \rho =Q_{\rm box} = {\bf n} \cdot [ {\bf D}_1 - {\bf D}_2 ] S$$ (39)

where vector ${\bf n}$ means the unit vector pointing from media 1 to 2.

$${\bf n} \cdot [ {\bf D}_1 - {\bf D}_2 ] = Q_{\rm box}/S = \sigma_{12}$$ (40)

From $\nabla \cdot {\bf B} = 0$

$${\bf n} \cdot [ {\bf B}_1 - {\bf B}_2 ] = 0$$ (41)

Stokes theorem: In Fig.1.3

$$\oint {\bf V}\cdot d{\bf r} = ({\rm I}) + ({\rm II}) + ({\rm III}) + ({\rm IV})$$

$$= V_x (x_0, y_0 )dx + V_y (x_0+dx, y_0 )dy + V_x (x_0 , y_0 + dy) (-dx) + V_y (x_0, y_0)(-dy)$$

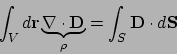
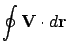
$$= V_x (x_0, y_0 )dx + [V_y (x_0, y_0 ) + \frac{\partial V_y}{\partial x}dx ]dy$$

$$- [V_x (x_0, y_0 ) + \frac{\partial V_x}{\partial y}dy ]dx - V_y (x_0, y_0 )dy$$

$$= \left( \frac{\partial V_y}{\partial x} - \frac{\partial V_x}{\partial y} \right) dx dy = ({\rm rot}{\bf V})_z dxdy$$

図 1: Gauss and Stokes box

図 2: Stokes theorem

From Eq.3

$$\oint_C {\bf E}\cdot d{\bf r} = \int_S {\rm rot}{\bf E}\cdot d{\bf S}$$ (42)

$$= - \int_S \frac{\partial {\bf B}}{\partial t}\cdot {\bf b} dS$$ (43)

$$\delta r [{\bf E}_1 \cdot {\bf t}_1 + {\bf E}_2 \cdot {\bf t}_2 ] = - \frac{\partial {\bf B}}{\partial t}\cdot {\bf b} \delta r \delta h \longrightarrow 0$$ (44)

$${\bf t} = {\bf t}_1 = -{\bf t}_2$$ (45)

$${\bf t}\cdot [{\bf E}_1 - {\bf E}_2 ] = 0$$ (46)

From Eq.4

$$\oint_C {\bf H}\cdot d{\bf r} = \int_S {\rm rot}{\bf H}\cdot d{\bf S}$$ (47)

$$= \int_S \left( {\bf J} + \frac{\partial {\bf D}}{\partial t}\right) \cdot {\bf b} dS$$ (48)

$$\delta r [{\bf H}_1 \cdot {\bf t}_1 + {\bf H}_2 \cdot {\bf t}_2 ] = \left( {\bf J} + \frac{\partial {\bf D}}{\partial t}\right) \cdot {\bf b} \delta r \delta h \longrightarrow {\bf J}\cdot{\bf b}\delta r \delta h$$ (49)

$${\bf t}\cdot [{\bf H}_1 - {\bf H}_2 ] = {\bf J}_s \ \ \ [\equiv {\rm surface \ current \ density} ({\rm Am}^{-1})]$$ (50)