If the light is reflected at a metal surface covered with a dielectric medium
(
![]() ) ,
e.g. with a BK7 half cylinder(n=1.515 at 633 nm) or SF10 glass(n=1.723 at
643.8 nm),
) ,
e.g. with a BK7 half cylinder(n=1.515 at 633 nm) or SF10 glass(n=1.723 at
643.8 nm),
| (197) |
| (198) |
The resonance condition of the light in the prism with the surface plasmon
at metal(1)|air(2) interface (Kretschmann-Raether configuration) is
| (199) | |||
 |
(200) |
The refelcted intensity ![]() can be given by Frensel's equations of the
prisim
can be given by Frensel's equations of the
prisim![]() metal
metal![]() air three layer system.
air three layer system.
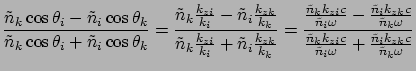 |
(201) | ||
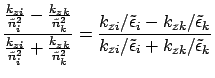 |
(202) | ||
| (203) |
For transmission
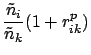 |
(204) | ||
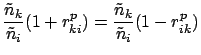 |
(205) | ||
| (206) |
The total reflection of the three layer model becomes
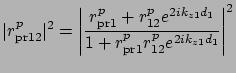 |
(207) | ||
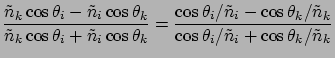 |
(208) | ||
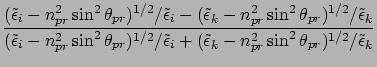 |
(209) | ||
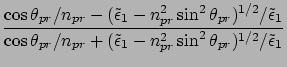 |
(210) |
The above equation for ![]() can be understood by
can be understood by
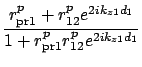 |
(211) | ||
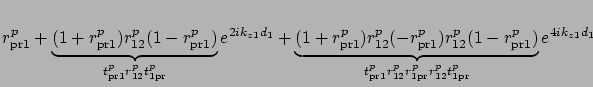 |
|||
| (212) | |||
| (213) | |||
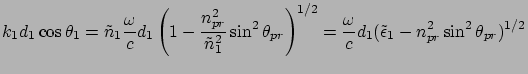 |
(214) |
The FORTRAN programs is
c234567-- spr_angle_3layer.f ---
c complex calculation
implicit real*8 (a-h,o-z)
complex*16 e1,rpr1,r12,rpr12,rpr12c,alpha,ref
complex*16 aaa,fukso,e2
c=2.99792458d8
hbar=6.5822d-16
pi=acos(-1.0d0)
c ------ air ----
e2=dcmplx(1.0d0,0.0d0)
c ----- SF10 633 nm
enpr=1.723d0
c ----- gold 633 nm
e1n=0.1726d0
e1k=3.4218d0
e1r=e1n**2-e1k**2
e1i=2.0d0*e1n*e1k
e1=dcmplx(e1r,e1i)
c ---- gold thickness (m)
d1=50.0d-9
c
ramd=633.0d-9
omega=2.0d0*pi/ramd*c
fukso=dcmplx(0.0d0,1.0d0)
write (6,*) ramd,omega,hbar*omega
c --------- angle scan ----------
ang0=35.0d0
ang1=45.0d0
do i=1, 1001
theta=(ang0+dble(i-1)/1000.0d0*(ang1-ang0))/180.0d0*pi
rpr1=(cos(theta)/enpr -
& sqrt(e1-enpr**2*sin(theta)**2)/e1)
& / (cos(theta)/enpr +
& sqrt(e1-enpr**2*sin(theta)**2)/e1)
r12=( sqrt(e1-enpr**2*sin(theta)**2)/e1 -
& sqrt(e2-enpr**2*sin(theta)**2)/e2 )
& / ( sqrt(e1-enpr**2*sin(theta)**2)/e1 +
& sqrt(e2-enpr**2*sin(theta)**2)/e2 )
aaa=2.0d0*omega/c*d1*sqrt(e1-enpr**2*sin(theta)**2)
alpha=aaa*fukso
rpr12=(rpr1+ r12*exp(alpha))/(1.0d0+rpr1*r12*exp(alpha))
rpr12c=conjg(rpr12)
ref=rpr12*rpr12c
write (6,*) theta/pi*180.0d0,dble(ref)
enddo
end
At resonance or ![]() the power of the SPs is lost by internal absorption
in the metal. This loss is compesated by the power of the incomig light. Both
have to be equal in the steady state.
the power of the SPs is lost by internal absorption
in the metal. This loss is compesated by the power of the incomig light. Both
have to be equal in the steady state.
If the reflectivity ![]() has lowest value, the intensity of the electromagnetic field
reaches its maximum in the surface. For 600 nm light
the maximum enhancement of the electric field intensity is ca. 200 for
silver film (60 nm thickness), 30 for gold film, 40 for aluminium film, and
7 for copper film, respectrively.
has lowest value, the intensity of the electromagnetic field
reaches its maximum in the surface. For 600 nm light
the maximum enhancement of the electric field intensity is ca. 200 for
silver film (60 nm thickness), 30 for gold film, 40 for aluminium film, and
7 for copper film, respectrively.